Post Your Answer
Post Your Answer
Post Your Answer
Post Your Answer
Ans 3:
Class : Class 4
Cannot Understand.SOF says that a. is the correct answer but a. has four pieces of the cube. In fact I think none are correct.

 (C)
(C)  (D)
(D) 
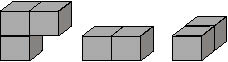
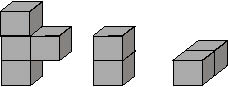
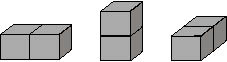
 The cube shown here was cut into three pieces. Which of the following groups could be the three pieces of the cube ?
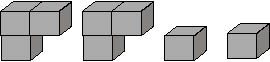
The cube shown here was cut into three pieces. Which of the following groups could be the three pieces of the cube ?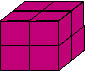
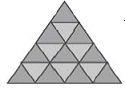
 Four pupils each made a statement about the given figure.
Four pupils each made a statement about the given figure.