Post Your Answer
Post Your Answer
Post Your Answer
Post Your Answer
Post Your Answer
Ans 2:
Class : Class 6
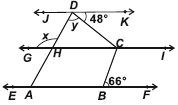
To solve this, let’s analyze the problem step-by-step:
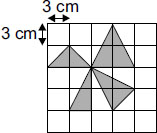
Find the area of a single small rectangle:
Each small rectangle has a length of 3 cm and a breadth of 2 cm.
So, the area of one small rectangle is:
3
cm
×
2
cm
=
6
sq. cm
3cm×2cm=6sq. cm
Calculate the total area of all 81 small rectangles in the big rectangle:
If there are 81 small rectangles, then the area of the big rectangle is:
81
×
6
sq. cm
=
486
sq. cm
81×6sq. cm=486sq. cm
Count the number of small rectangles remaining in the figure:
From the image, we can count the number of small rectangles remaining. By visually inspecting, there appear to be 62 small rectangles left.
Calculate the area of the remaining rectangles:
With 62 rectangles remaining, the area of the remaining part is:
62
×
6
sq. cm
=
372
sq. cm
62×6sq. cm=372sq. cm
Find the total area of the rectangles removed:
Subtract the area of the remaining rectangles from the total area to find the area of the removed rectangles:
486
sq. cm
−
372
sq. cm
=
114
sq. cm
486sq. cm−372sq. cm=114sq. cm
Thus, the total area of the rectangles removed is 114 sq. cm.
The correct answer is B) 114 sq. cm.
Ans 6:
Class : Class 8
can you please tell me how you got 19. Harshit Solanki from Dps, Indore.area of 1 small rectangle = 3*2 =6 sq. cm.Total cards removed = 19 so ,19*6= 114 sq. cm.
Ans 20:
Class : Class 7
bro the small square at the is an rectangle and the thing is 2 rectangle so 19 square(rectangle) are there
Ans 24:
Class : Class 6
area of 1 small rectangle = 3*2 =6 sq. cm.Total cards removed = 19 so ,19*6= 114 sq. cm.
Ans 28:
Class : Class 8
I can explain those squares you see? sof refers to them by saying small rectangles. 5are in moth